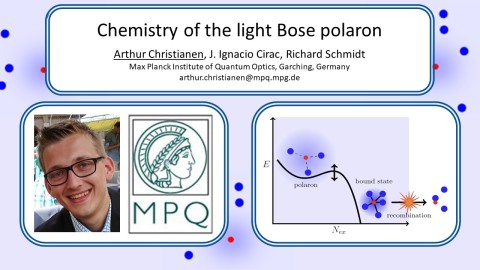
Chemistry of the light Bose polaron

In ultracold atomic gases, a unique interplay arises between condensed matter physics, few-body physics and chemistry. We study how a typical condensed matter phenomenon: quasiparticle dressing of an impurity, can modify its few-body physics and chemical reactivity. Using a Gaussian state variational method in the frame of the impurity, we demonstrate that three-body correlations lead to an instability of the polaron. This instability is connected to a three-body scattering resonance, but shifted to smaller interactions, showing that polaron formation stimulates the associated chemical processes.

Authors: Arthur Christianen, J. Ignacio Cirac, Richard Schmidt

Affiliations: Max Planck Institute of Quantum Optics

Disorder-free many-body localization in 1D and 2D

We consider interacting particles under the influence of a constant potential gradient, corresponding to Wannier-Stark localization in the noninteracting limit, on both a one-(1) and two-dimensional lattice. The eigenstate thermalization hypothesis in its strong formulation is violated even for arbitrarily weak tilts. Further, there is area-law rather than logarithmic growth of entanglement. This type of localization is therefore essentially different from disorder-driven many-body localization. (1) Elmer V. H. Doggen, Igor V. Gornyi, Dmitry G. Polyakov, Phys. Rev. B 103, L100202 (2021)

Authors: Elmer V. H. Doggen (1)(2), Igor V. Gornyi (1)(2)(3)(4)(5), Dmitry G. Polyakov (1)

Affiliations: 1) Institute for Quantum Materials and Technologies, Karlsruhe Institute of Technology, 76021 Karlsruhe, Germany (2) Institut für Theorie der Kondensierten Materie, Karlsruhe Institute of Technology, 76128 Karlsruhe, Germany (3) A. F. Ioffe Physico-Technical Institute, 194021 St. Petersburg, Russia (4) L. D. Landau Institute for Theoretical Physics RAS, 119334 Moscow, Russia (5) Petersburg Nuclear Physics Institute, 188300 St. Petersburg, Russia

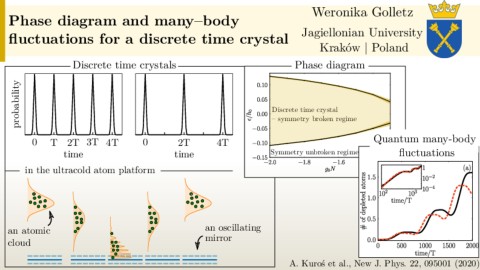
Phase diagram and many–body fluctuations for a discrete time crystal

Spontaneous symmetry breaking is a fundamental concept in many areas of physics. The space crystals, superconductors and ferromagnets are respective examples of continuous space translation, gauge and rotational invariance breaking. Despite its popularity, the idea of breaking the continuous time translation symmetry and the discrete time-translation symmetry (DTTS) has received attention only very recently and manifested in the form of discrete time crystals (1-3). It was shown that isolated periodically driven ultracold atoms (2) are able to spontaneously self--reorganise their motion leading to DTTS breaking. Here we focus on these kinds of systems bouncing resonantly on an oscillating atom mirror with the interaction between atoms greater than a critical value. Such a driven cloud of ultracold atoms moves with a period n-times longer than that of the mirror due to DTTS breaking, and the so-called n-tupling discrete time crystal (nDTC) is formed. These systems are promising for experimental realisation (4) As the experimental conditions are never perfect, we analyse the robustness of nDTC against small perturbations of the initial state by determining the phase diagrams. Moreover, we investigate the quantum many-body fluctuations of nDTCs resulting from interactions between atoms within the Bogoliubov theory and demonstrate that DTCs are resistant to quantum many-body effects (5). References (1) F. Wilczek, Phys. Rev. Lett. 109, 160401 (2012) (2) K. Sacha, Phys. Rev. A 91, 033617 (2015) (3) D. Else, et al., Phys. Rev. Lett 117, 090402 (2016) (4) K. Giergiel et al., New J. Phys. 22, 085004 (2020) (5) A. Kuroś et al., New J. Phys. 22, 095001 (2020)

Authors: Arkadiusz Kuros (1), Rick Mukherjee (2), Weronika Golletz (1), Frederic Sauvage (2), Krzysztof Giergiel (1), Florian Mintert (2), and Krzysztof Sacha (1)

Affiliations: (1) Institute of Theoretical Physics, Jagiellonian University in Cracow, Poland (2) Blackett Laboratory, Imperial College London, SW7 2AZ, UK

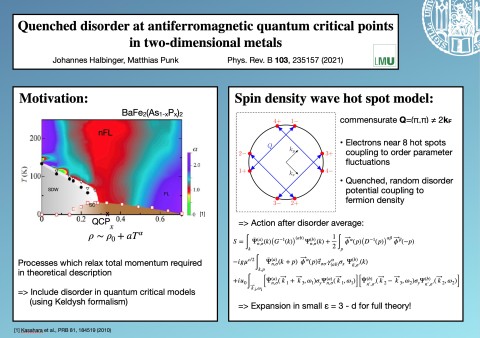
Quenched disorder at antiferromagnetic quantum critical points in two-dimensional metals

We study spin density wave quantum critical points in two dimensional metals with a quenched disorder potential coupling to the electron density. Adopting an ε-expansion around three spatial dimensions, where both disorder and the Yukawa-type interaction between electrons and bosonic order parameter fluctuations are marginal, we present a perturbative, one-loop renormalization group analysis of this problem, where the interplay between fermionic and bosonic excitations is fully incorporated. Considering two different Gaussian disorder models restricted to small-angle scattering, we show that the non-Fermi liquid fixed point of the clean SDW hot spot model is generically unstable and the theory flows to strong coupling due to a mutual enhancement of interactions and disorder. We study properties of the asymptotic flow towards strong coupling, where our perturbative approach eventually breaks down. Our results indicate that disorder dominates at low energies, suggesting that the ground-state in two dimensions is Anderson-localized.

Authors: Johannes Halbinger and Matthias Punk

Affiliations: Physics Department, Arnold Sommerfeld Center for Theoretical Physics, Center for NanoScience, and Munich Center for Quantum Science and Technology (MCQST), Ludwig-Maximilians University Munich

An Operational Definition of Topological Order

Topological phases of matter are beyond the conventional Landau symmetry breaking principle. Since their discovery, these phases of matter have been identified by indirect signatures rather than direct measurable quantitites. Additionally, some important cases like topological order of mixed quantum states still remains unaddressed. Topological error correction being inherently related to these phases, we aim to recast the notion of topological order in terms of the error correction ability of a given system to address the above issues.

Authors: Amit Jamadagni, Hendrik Weimer

Affiliations: Leibniz University, Hannover

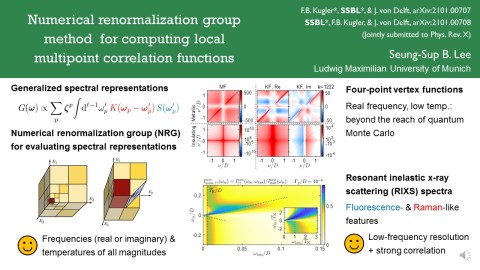
Numerical renormalization group method for computing local multipoint correlation functions

Multipoint correlation functions on the real-frequency axis describe experimentally relevant properties, such as nonlocal susceptibilities, transport, and inelastic photon scattering, of the systems of interacting quantum particles. However, the non-perturbative computation of real-frequency multipoint functions has been intractable. In my poster, I will summarize our recently developed method for non-perturbatively computing multipoint functions and its applications [1, 2]. We have derived generalized spectral representations for multipoint functions that apply in all of the commonly used many-body frameworks [1]: the imaginary-frequency Matsubara and the real-frequency zero-temperature and Keldysh formalisms. We have developed a numerical renormalization group (NRG) method for evaluating the spectral representations for local multipoint functions, which can treat temperatures and frequencies---imaginary or real---of all magnitudes, from large to arbitrarily small ones [2]. I will present the numerical results of four-point vertex functions and resonant inelastic x-ray scattering (RIXS) spectra of quantum impurity systems.
[1] F. B. Kugler*, S.-S. B. Lee*, and J. von Delft, arXiv:2101.00707, Accepted in Phys. Rev. X.
[2] S.-S. B. Lee*, F. B. Kugler, and J. von Delft, arXiv:2101.00708, Accepted in Phys. Rev. X.
*: (co-)first author(s).

Authors: Seung-Sup B. Lee (1), Fabian B. Kugler (1,2), and Jan von Delft (1)

Affiliations: 1: Ludwig Maximilian University of Munich, Germany. 2: Rutgers University, USA

Chiral Numerical Renormalization Group (NRG) for Quantum Impurities Coupled to Topological Edge Modes

The Kondo effect arises when an electronic channel interacts with a quantum impurity, e.g., a localized spin, forming a coherent quantum many-body screening cloud around it. In its multi-channel variants, the Kondo effect exhibits even more exotic non-Fermi-liquid behavior, as well as fractionalized excitations (anyons) pinned to the impurities. Intriguing physics also occurs when multiple impurities are coupled to the same channel(s), especially when these channel(s) are chiral, i.e., allow electrons to propagate only in one direction, as on the edge of quantum hall systems. Lopes et al. [Phys. Rev. B 101, 085141 (2020)] propose that in such a system, the emerging anyons can be exploited for topological quantum computation. Understanding the opportunities offered by such setups requires their detailed numerical modeling. The standard simulation tool for the study of quantum impurities is Wilson’s numerical renormalization group (NRG). A key part in it is mapping the electronic bath into a so-called Wilson chain – a tight-binding chain with the impurity at one edge and hopping amplitudes decaying exponentially with the distance. However, lattice models are typically incompatible with chirality, and indeed this mapping breaks down. In our work we bridge this gap by developing a new chiral version of NRG. For this we map the bath to a new geometry, a “Wilson ladder”. Using chiral NRG and analytical arguments we find that the breaking of time-reversal symmetry, due to chirality, allows the appearance of effective chiral-Kondo terms in the Hamiltonian, which have not been previously considered. The “Wilson ladder” construction lets us probe the interplay between the Kondo screening length and the finite distance between the impurities in order to characterize these chiral-Kondo terms, as well other exotic phenomena which might arise.

Authors: Matan Lotem, Eran Sela, and Moshe Goldstein

Affiliations: Tel Aviv University, Tel Aviv, Israel

Signatures of phase transitions and optical bistability for atoms in optical lattices

Radiative dipole-dipole interactions lead to a rich phenomenology of phases in an optical lattice of atoms when driven by a coherent field. They can also support intrinsic optical bistability, where it is possible for two such phases to coexist. Here, we investigate the different phases that emerge in an array of atoms and how they can be identified by studying the scattered light. We find that the onset of phases predicted by mean-field theory are revealed by large jumps in coherent and incoherent signals of the transmitted light. The presence of bistability can result in a strong cooperative and weak single-atom response from the array, with hysteresis upon sweeping the incident light frequency. We discuss how the phases depend on the low light intensity collective modes, and also determine the thresholds for the optical bistability in terms of the atomic cooperativity parameter and intensity of the incident light, in many cases obtaining analytical results.

Authors: C. D. Parmee (1), J. Ruostekoski (1)

Affiliations: (1) Lancaster University

Identifying Majorana vortex modes via non-local transport

We propose a detection scheme for Majorana vortex modes based on non-local quantum transport.

Authors: Björn Sbierski, Max Geier, Rob Moore, Joel Moore

Tailored coexistence of topological and nontopological Fermi superfluid phases

The two-dimensional spin-imbalanced Fermi gas subject to s-wave pairing and spin-orbit coupling is considered a promising platform for realizing a topological chiral-p-wave superfluid. In the BCS limit of s-wave pairing, i.e., when Cooper pairs are only weakly bound, the system enters the topological phase via a second-order transition driven by increasing the Zeeman spin-splitting energy. For larger Cooper-pair bound-state energies, the system undergoes the BCS-BEC crossover, in the course of which the topological transition becomes first-order. As a result, topological and nontopological superfluids coexist in spatially separated domains in an extended region of phase space spanned by the strength of s-wave interactions and the Zeeman energy. Here we investigate this phase-coexistence region theoretically using a zero-temperature mean-field approach. The internal interface between topological and nontopological parts of the system is found to host unconventional quasiparticle excitations - including a Majorana bound state as expected based on topology - whose properties can be tailored by tuning parameters that are routinely accessible in ultracold-atom experiments. As part of our work, we present general theoretical insights into the properties of Andreev bound states at interfaces between two superfluids with different pair-potential magnitudes. In particular, such asymmetric junctions are found to support Andreev bound states only within a restricted range for the pair-potential phase difference across the interface.

Authors: (1) Victoria University of Wellington, New Zealand (2) Massey University, New Zealand

Affiliations: Kadin Thompson (1), Uli Zuelicke (1), Joachim Brand (2)

Structural and Electronic Properties of the FeSi(110) Surface

Iron silicide (FeSi) is a fascinating material which has attracted numerous research efforts for decades[1]. It has B20 crystal structure featuring cubic unit cell without an inversion center. FeSi is a small-band-gap semiconductor with correlated electronic characteristics, closely resembling to the Kondo insulators whereby a coherent picture of intrinsic properties and underlying physics remains to be fully developed. To gain further insight into the unusual surface properties of this system, we investigated atomically flat FeSi(110) surface using scanning tunneling microscopy/spectroscopy (STM/STS) as well as density functional theory modeling. A distinct asymmetric electronic gap of 73 meV close to the Fermi level has been resolved. Intriguingly, a residual density of states and two in-gap states have been identified in the gap regime for the first time. These findings support and provide further insight into the emergence of surface metallicity in the low-temperature regime.[2] [1] V. Jaccarino, G. K. Wertheim, J. H. Wernick, L. R. Walker, S. Arajs. Phys. Rev. 1967, 160, 476. [2] B. Yang, M. Uphoff, Y.-Q. Zhang, et al. Proc. Natl. Acad. Sci. 2021, 118, e2021203118.

Authors: Biao Yang,†,1 Martin Uphoff,†,1 Yi-Qi Zhang,†,1,* Joachim Reichert,1 Ari P. Seitsonen,2 Andreas Bauer,3 Christian Pfleiderer,3 Johannes V. Barth1,*

Affiliations: Q1 Physics Department E20, Technical University of Munich, D-85748 Garching, Germany 2 Département de Chimie, École Normale Supréieure, 24 rue Lhomond, F-75005 Paris, France 3 Physics Department E51, Technical University of Munich, D-85748 Garching, Germany

Impact of domian disorder on the optoelectronic properties of semimetal MoTe2

Condensation signatures of photogenerated interlayer excitons in a van der Waals heterostack

We optically investigate interlayer excitons in TMDC heterostacks at cryogenic temperatures and observe several criticalities with respect to photoluminescence intensity, linewidth and temporal coherence which point towards the condensation of the photogenerated excitons. The phenomena survive above 10 K , consistent with the predicted critical condensation temperature. Our study provides a first phase-diagram of many-body interlayer exciton states.

Authors: Lukas Sigl (1,2), Florian Sigger (1,2), Mirco Troue (1,2), Fabian Kronowetter (1), Jonas Kiemle (1,2), Julian Klein (1), Jonathan J. Finley (1,2), Ursula Wurstbauer (1,3) and Alexander W. Holleitner (1,2)

Affiliations: Walter Schottky Institut - Zentrum für Nanotechnologie und Nanomaterialien and Physik-Department, TU Munich, Am Coulombwall 4, 85748 Garching, Germany Munich Center for Quantum Science and Technology (MCQST), Schellingstr. 4, 80799 München, Germany Institute of Physics, Westfälische Wilhems-Univerität Münster, Wilhelm-Klemm-Str. 10, 48149 Münster, Germany